Answer:
Option A.
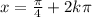 and
and
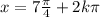
Explanation:
We have the following expression:
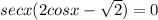
For this equation to fulfill any of the two terms, or both, they must be zero:
That is to say:
 or
or
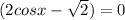
We know that
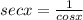 is different from 0 for all x.
is different from 0 for all x.
Then we only have one option left.
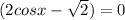
We clear x from the equation:
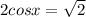
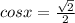
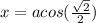
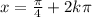 and
and
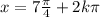
where k is an integer