Answer:
The answer is indeed, 85/12 ; B
Using the Pythagorean theorem, you can use FOIL to solve for the hypotenuse then subtract the radius from the entire hypotenuse to find R. Since all radii are congruent.
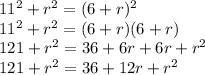
Simplify the rest. We also know that (6+r)^2 is the hypotenuse since angle JKL is a right angle as it is perpendicular to the center and is a tangent.