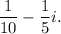Answer: The required simplified quotient is
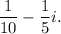
Step-by-step explanation: We are given to find the following quotient :
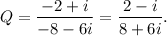
To find the given quotient, we must multiply both the numerator and denominator by the conjugate of (8+6i), that is, (8-6i).
So, we have
![Q\\\\\\=(2-i)/(8+6i)\\\\\\=((2-i)(8-6i))/((8+6i)(8-6i))\\\\\\=(16-12i-8i+6i^2)/(64-36i^2)\\\\\\=(16-20i-6)/(64+36)~~~~~~~~~~~~~~~~~~[\textup{since }i^2=-1]\\\\\\=(10-20i)/(100)\\\\\\=(1-2i)/(10)\\\\\\=(1)/(10)-(1)/(5)i.](https://img.qammunity.org/2020/formulas/mathematics/middle-school/sxeaoqxm9l0peurq6ml4ui1mo6zxa4x6j9.png)
Thus, the required simplified quotient is