Question

How to change from expo to radical form

Answer 1

This one is easy if you remember that

`√(5) = {5}^{ (1)/(2) } \\ \sqrt[3]{5} = {5}^{ (1)/(3) } \\ \sqrt[4]{5} = {5}^{ (1)/(4) } \\ \sqrt[5]{5} = {5}^{ (1)/(5) }`

It keeps going. The bottom number in an exponential is the one on the outside of the radical.

To remember this:

The top number in the exponential is top dog, he get to go inside the radical.

The bottom number is not top dog, so he has to be outside the radical.

`\sqrt[3]{{5}^(7) } = {5}^{ (7)/(5) } \\ \sqrt[9]{{5}^(4) } = {5}^{ (4)/(9) }`

So for problem 4)

`{(32x)}^{ (1)/(2) } = √(32x)`

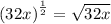
Because 1/2 is the expo form of a normal square root radical.

Problem 3)

`{(2x)}^{ (5)/(6) } = \sqrt[6]{ {2x}^( 5 ) }`

I should say

`√(5) = \sqrt[2]{5}`

However, the 2 is Normally never stated. You only plave a number on the front of the radical if it is larger than 2. Normally.

Additionally: it technically looks like this, but both the 2 and the 1 are not normally written. Because 5^1 = 5

`{5}^{ (1)/(2) } = \sqrt[2]{ {5}^(1) }`

Remember on your expo to radical, if its

`{(\sqrt[3]{5} )}^{ (8)/(6) } = {5}^{ (1)/(3) * (8)/(6) } = {5}^{ (4)/(9) } = \sqrt[9]{ {5}^(4) }`

Because when you have an exponant raised to an exponant with the same base number, just multiply them and keep the base, like the above example.

If you have more questions, let me know.

Answer 2

See below.

Explanation:

If the exponent is a/b then a is the power and b is the value of the radical.

Example: x^2/3 = ∛x². 2 is the power and 3 is the radical.

Answers:

1) 7^2/3 = ∛7²

5) √3 = 3^1/2

9) (∛27)⁴ = 27^4/3 = 3^4 = 81.