Question

Use symmetry to graph the inverse of the function.

Answer 1

The correct option is a.

Explanation:

If a function is defined as

`f(x)=\{(x,y):x\in R,y\in R\}`

then the inverse of the function is

`f^(-1)(x)=\{(y,x):x\in R,y\in R\}`

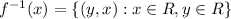
We know that the graph of a function and its inverse function symmetrical about the line y=x.

Draw the line of symmetry x=y in each of the given graph.

From the below figure it is clear that only graph 1 is symmetrical about the line y=x.

Therefore the correct option is a.