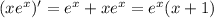Use first principle to find y'all for y=xe^x

Question

asked Jun 14, 2020 89.5k views

1 Answer

Peshkira · Answer 1 · 2020-06-18T01:57:34+0000

By definition of the derivative,

$y'=\displaystyle\lim_(h\to0)\frac{(x+h)e^(x+h)-xe^x}h$

We can employ the standard manipulation for proving the product rule:

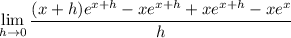
$\displaystyle\lim_(h\to0)\frac{(x+h)e^(x+h)-xe^(x+h)+xe^(x+h)-xe^x}h$

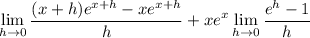
$\displaystyle\lim_(h\to0)\frac{(x+h)e^(x+h)-xe^(x+h)}h+xe^x\lim_(h\to0)\frac{e^h-1}h$

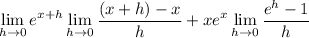
$\displaystyle\lim_(h\to0)e^(x+h)\lim_(h\to0)\frac{(x+h)-x}h+xe^x\lim_(h\to0)\frac{e^h-1}h$

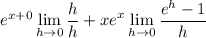
$e^(x+0)\displaystyle\lim_(h\to0)\frac hh+xe^x\lim_(h\to0)\frac{e^h-1}h$

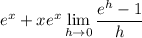
$e^x+xe^x\displaystyle\lim_(h\to0)\frac{e^h-1}h$

The remaining limit is pretty well-known and has a value of 1. We can derive it from the definition of
,

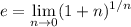
$e=\displaystyle\lim_(n\to0)(1+n)^(1/n)$

In the limit above, we substitute
$\eta=e^h-1$ , so that
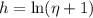$h=\ln(\eta+1)$ . As
$h\to0$ , we have
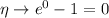$\eta\to e^0-1=0$ :

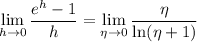
$\displaystyle\lim_(h\to0)\frac{e^h-1}h=\lim_(\eta\to0)\frac\eta{\ln(\eta+1)}$

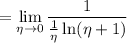
$\displaystyle=\lim_(\eta\to0)\frac1{\frac1\eta\ln(\eta+1)}$

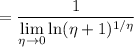
$\displaystyle=\frac1{\lim\limits_(\eta\to0)\ln(\eta+1)^(1/\eta)}$

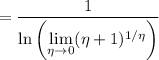
$\displaystyle=\frac1{\ln\left(\lim\limits_(\eta\to0)(\eta+1)^(1/\eta)\right)}$

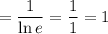
$=\frac1{\ln e}=\frac11=1$

After all this, we've shown that

(xe^x)'=e^x+xe^x=e^x(x+1)