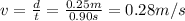The average time that it takes for the car to travel the first 0.25m is 2.23 s
The average time that it takes for the car to travel the first 0.25 m is given by:
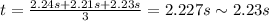
The average time to travel just between 0.25 m and 0.50 m is 0.90 s
First of all, we need to calculate the time the car takes in each trial to travel between 0.25 m and 0.50 m:
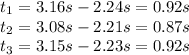
Then, the average time can be calculated as

Given the time taken to travel the second 0.25 m section, the velocity would be 0.28 m/s
The velocity of the car while travelling the second 0.25 m section is equal to the distance covered (0.25 m) divided by the average time (0.90 s):