Question

Write an equation in slope-intercept form that is parallel to the line 3x + 2y = 1 and passes through the point (1, 0). A)

y = 3/ 2 x + 2 B) y = − 2 /3 x + 1 C) y = − 2/3 x - 1 D) y = − 3/2 x + 3 /2

Answer 1

D.
`y=-(3)/(2) x+(3)/(2)`

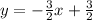
Explanation:

First we need to find the slope of the line of 3x + 2y = 1 because the line is parallel to this line.

Find the slope of 3x + 2y = 1 by making the equation equal to y. Subtract 3x from both sides of the equation.

• 2y = 1 - 3x

Divide both sides by 2 to isolate and solve for y.

• y = 1/2 - 3/2x

The slope of the line is the coefficient of x, so the slope of the line is -3/2.

Now we have the slope of the line and a point that the line passes through, so we can substitute these values into point-slope form.

Point-slope form:
`y-y_1=m(x-x_1)`

Substitute -3/2 for m and the point (1,0) into
`x_1` and
`y_1`.

• 
  `y-(0)=-(3)/(2) (x-1)`

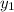Distribute -3/2 inside the parentheses.

• 
  `y=-(3)/(2) x+(3)/(2)`

This is the final answer in slope-intercept form (y = mx + b).

Answer 2

D) y = − 3/2 x + 3 /2

Explanation:

3x+2y =1

We need to get this in slope intercept form (solve for y)

Subtract 3x from each side

3x-3x +2y = -3x+1

2y = -3x+1

Divide by 2

2y/2 = -3/2x+1/2

The slope is -3/2

We want a line that is parallel so the slope is the same

m = -3/2

We an use point slope form since we have the slope and a point

y - y1 = m(x-x2)

y - 0 = -3/2(x-1)

Distribute

y = -3/2x +3/2