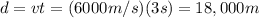Question

Equations to use: v= λ ∙ f v=d/t

b. A guitar string has a length of 0.90 m. When you pluck it, it plays a “C” that has a frequency of 256 Hz. How fast is the wave moving back and forth along the string? (

c. In a train track made of solid steel, sound waves travel extremely fast – about 6,000 m/s. If a train’s vibration travels for 3 seconds, how far will it move?

Answer 1

b. 460.8 m/s

Step-by-step explanation:

We have a stringth with length L = 0.90 m, and a standing wave with frequency f = 256 Hz. The equation that relates the speed of the wave with the frequency and the length of the string is

`f=(v)/(2L)`

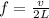
where v is the speed of the wave. Re-arranging the equation and putting in the numbers, we find:

`v=2Lf=2(0.90 m)(256 Hz)=460.8 m/s`

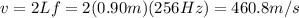
c. 18,000 m

Step-by-step explanation:

The speed of the sound wave in steel is v = 6000 m/s. The train's vibration travels for 3 seconds, so the distance covered by the sound wave during this time can be calculated using the formula

`v=(d)/(t)`

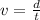
Re-arranging the equation and substituting the numbers, we can find d:

`d=vt=(6000 m/s)(3 s)=18,000 m`

Answer 2

b. The guitar represents half of the wave length. So the full wave length is 2x0.9m = 1.8m.

Using the given equation, v= λ ∙ f,

the wave is moving back and forth along the string at 1.8 ∙ 256

= 460.8m/s

c. Sound waves travel at 6,000 m/s.

Using the given equation, v=d/t, or d=vt,

train's vibration in 3 seconds travels 6000*3

=18,000m or 18km