Question

Simplify the expression 3x(x-12x)+3x^2-2(x-2)^2.which statements Are true about the process and simplified product check all that apply

Answer 1

-8 (4 x^2 - x + 1)

Explanation:

Simplify the following:

3 x (x - 12 x) + 3 x^2 - 2 (x - 2)^2

x - 12 x = -11 x:

3 x×-11 x + 3 x^2 - 2 (x - 2)^2

3 x (-11) x = 3 x^2 (-11):

-11×3 x^2 + 3 x^2 - 2 (x - 2)^2

3 (-11) = -33:

-33 x^2 + 3 x^2 - 2 (x - 2)^2

3 x^2 - 33 x^2 = -30 x^2:

-30 x^2 - 2 (x - 2)^2

(x - 2) (x - 2) = (x) (x) + (x) (-2) + (-2) (x) + (-2) (-2) = x^2 - 2 x - 2 x + 4 = x^2 - 4 x + 4:

-30 x^2 - 2 x^2 - 4 x + 4

-2 (x^2 - 4 x + 4) = -2 x^2 + 8 x - 8:

-2 x^2 + 8 x - 8 - 30 x^2

Grouping like terms, -2 x^2 - 30 x^2 + 8 x - 8 = (-30 x^2 - 2 x^2) + 8 x - 8:

(-30 x^2 - 2 x^2) + 8 x - 8

-30 x^2 - 2 x^2 = -32 x^2:

-32 x^2 + 8 x - 8

Factor -8 out of -32 x^2 + 8 x - 8:

Answer: -8 (4 x^2 - x + 1)

Answer 2

The final product of the expression when simplified is: -30x² + 8x - 8. Thus, statements C and D are true.

How to simplify an expression?

The given expression is
`\(3x(x - 12x) + 3x^2 - 2(x - 2)^2\)`.

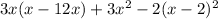a. The term -2(x - 2)² is simplified by first squaring the expression x - 2 as shown in the calculation below:

-2(x - 2)² = -2(x² - 4x + 4) = -2x² + 8x - 8

b. The simplified product is not a binomial; it's a trinomial.

c. After multiplying, the like terms are combined by adding and subtracting as demonstrated below:

Now let's substitute the simplified form of -2(x - 2)² back into the expression:

3x(x - 12x) + 3x² - 2(x - 2)² = 3x(-11x) + 3x² - 2x² + 8x - 8.

-33x² + 3x² + 8x - 8 = -30x² + 8x - 8.

d. As demonstrated above, we see that the parentheses are eliminated through multiplication.

e. The final simplified product is -30x² + 8x - 8, not -28x² + 8x - 8, so statement e is false.

Complete Question:

Simplify the expression 3x(x – 12x) + 3x2 – 2(x – 2)2. Which statements are true about the process and simplified product? Check all that apply.

a. The term –2(x – 2)2 is simplified by first squaring the expression x – 2.

b. The simplified product is a binomial.

c. After multiplying, the like terms are combined by adding and subtracting.

d. The parentheses are eliminated through multiplication.

e. The final simplified product is –28x2 +8x – 8.