Answer:
The correct options are A and C.
Explanation:
The given functions are
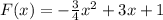
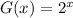
We have to find to solution for the equation
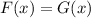
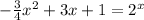
The solution of the above equation is the intersection of F(x) and G(x).
From the graph it is noticed that both graph intersects each other at (0,1) and (2,4).
The x-coordinates of these points are the solutions of the given equation.
Therefore the solution of given equation are 0 and 2.
Therefore option A and C are correct.