Question

Find the equation of the line that is perpendicular to 5y=-4x , passing through (12,-4) . Express the answer the standard form...

HELP ME PLEASE.

Answer 1

The equation is 5x - 4y = 76

Explanation:

∵ The equation of the line 5y = -4x⇒ divide each side by 5

∴ y = -4/5 x ⇒ the slope of this line =
`(-4)/(5)`

∵ The two lines are ⊥

∴ The product of their slopes = -1

∴ The slope of the ⊥ line is
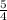`(5)/(4)` (additive and multiplicative inverse of the first slope)

∵ (y - y1) = m (x - x1) ⇒ m is the slope and (x1 , y1) point on the line

∴ y - (-4) = 5/4 (x - 12)

∴ y + 4 = 5/4 x - 15 ⇒ multiply both side by 4

∴ 4y + 16 = 5x - 60 ⇒ collect like terms

∴ 5x - 4y = 76

∴ The equation of the ⊥ line in standard form is 5x - 4y = 76