2 Answers

Prefect · Answer 1 · 2020-04-01T07:27:07+0000

Answer:

When original wave superimpose with its own reflected wave travelling in opposite direction then it will produce standing wave

Step-by-step explanation:

Let say the equation of original wave is

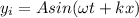
$y_i = A sin(\omega t + kx)$

now the equation of its reflected wave which is reflected 100% is given as

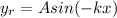
$y_r = A sin(\omegat - kx)$

now by superposition of above two waves we will have

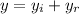
y = y_i + y_r

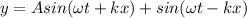
$y = A { sin(\omega t + kx) + sin(\omega t - kx)}$

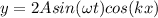
$y = 2Asin(\omega t)cos(kx)$

so above shows the equation of standing wave

so we can say that When original wave superimpose with its own reflected wave travelling in opposite direction then it will produce standing wave

Please log in or register to add a comment.