Question

Mr. Martin’s math test, which is worth 100 points, has 29 problems. Each problem is worth either 5 points or 2 points. Let x be the number of questions worth 5 points and let y be the number of questions worth 2 points. x + y = 29, 5x + 2y = 100 How many problems of each point value are on the test? 10 problems worth 5 points and 25 problems worth 2 points 14 problems worth 5 points and 15 problems worth 2 points 16 problems worth 5 points and 13 problems worth 2 points 15 problems worth 5 points and 13 problems worth 2 points

Answer 1

14 problems worth 5 points and 15 problems worth 2 points

14 x 5 = 70

15 x 2= 30

70 + 30= 100

14+15 = 29 5 x 14 + 2 x 15=100

Answer 2

Option 2.

Explanation:

Let x be the number of questions worth 5 points and let y be the number of questions worth 2 points.

Mr. Martin’s math test, which is worth 100 points, has 29 problems.

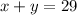`x+y=29` .... (1)

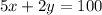`5x+2y=100` ..... (2)

Multiply equation (1) by 2.

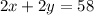`2x+2y=58` .... (3)

Subtract equation (3) from equation (2), to eliminate the variable y.

`5x+2y-(2x+2y)=100-58`

`5x+2y-2x-2y=42`

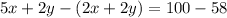
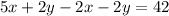
Combined like terms.

`(5x-2x)+(2y-2y)=42`

`3x=42`

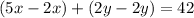
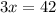
Divide both sides by 3.

`x=14`

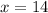
The value of x is 14.

Substitute x=14 in equation (1).

`14+y=29`

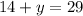
Subtract 14 from both sides.

`y=29-14`

`y=15`

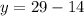
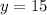
The value of y is 15.

It means 14 problems worth 5 points and 15 problems worth 2 points.

Therefore, the correct option is 2.