Question

In any function, f(x) = a(x-h)n + k , a negative leading coefficient means the graph is reflected.

True or False?

Answer 1

True

Explanation:

For any function of the form:

`f(x)=a(x-h)^n+k`

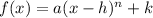
The coefficient
`a` produces a transformation called a reflection in the x-axis whenever
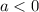`a<0`, that is, this axis is said to be a mirror. Let's take an example, suppose you have a function:

`f(x)=4(x-2)^2+5`

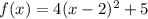
If the leading coefficient is now -4, then the graph is reflected and the new function is:

`f(x)=-4(x-2)^2+5`

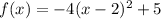
This is shown in the figure below. The red parabola represents
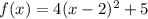`f(x)=4(x-2)^2+5` and the blue parabola is
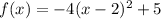`f(x)=-4(x-2)^2+5`. As you can see, the red parabola has been reflected and transformed into the blue one.