Question

Part 1: Label points A, B, and C on the triangle.

Given: In ΔABC, DE is parallel to AC.

Part 2: Use the spaces provides below to complete a formal proof.
Given: In ΔABC, DE is parallel to AC
Prove:
`(DB)/(BA) = (BE)/(BC)`

Answer 1

Explanation:

Find in attached file the triangle ABC with vertices labelled. Also a line DE parallel to AC

To prove that sides of DBE and CBA are proportional.

Since DE is parallel to AC we have

angle D=angle C

angle E = angle A (because of corresponding angles postulate)

Angle B =angle B(reflexive property)

Since all angles are congruent the triangles DBE and CBA are similar

Hence corresponding sides are proportional

i.e.

`(DB)/(BA) =(BE)/(BC)`

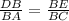
thus proved

Answer 2

Explanation:

Given parameters: In these two triangles side AC║DE and angle A is common in both the triangles.

We have to prove
`(DE)/(BE)=(BE)/(BC)`

As we know in two triangles if two angles are same then by AA theorem the triangles will be similar.

Since AC║DE and AB is a transverse.

Therefore ∠BDE=∠BAC

Similarly AC║DE and BC is a transverse

Therefore ∠BED=∠BCA

Here AA theorem fulfills the requirements of congruence in ΔABC≅ ΔBDE

Therefore all sides of these triangles will be in same ratio.

`(DB)/(BA) = (BE)/(BC)`