Answer:
S.A = 704 cm^2
Explanation:
We know that the formula to find the surface area of a cone:
S.A =
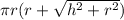 , where "r" is the radius and "h" is the height.
, where "r" is the radius and "h" is the height.
Given: r = 7 cm and h = 24 cm. The value of π = 22/7
Now plug in the given values in the above formula, we get
S.A =
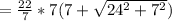
S.A =
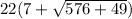
S.A =
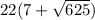
S.A = 22( 7 + 25)
S.A = 22(32)
S.A = 704 cm^2
The surface area of the cone is S.A = 704 cm^2.