Answer:

The value of r is 0.451
The percentage decrease is about 45.1%
Explanation:
The given exponential function is
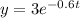
Using the exponent rule,
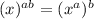
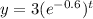
Now the value of
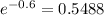
Thus, we can rewrite the function as
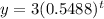
Therefore, the function in form
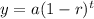

The value of r is 0.451
The percentage decrease is about 0.451 ×100 = 45.1%