Answer:
Option 1 , Option 2 and Option 4 are geometric sequence.
Explanation:
A sequence is said to be geometric when their exist a common ratio between the consecutive terms.
Option 1) -2.7,-9,-30,-100...
Common ratio=

=

=
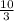
Common ratio=
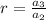
=
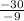
=
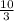
Common ratio=

=
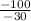
=
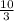
Since the common ratios are equal .
Thus -2.7,-9,-30,-100... is a geometric sequence .
Option 2) -1,2.5, -6.25, 15.625 ...
Common ratio=

=
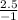
=

Common ratio=
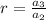
=

=

Common ratio=

=

=

Since the common ratios are equal .
Thus -1,2.5, -6.25, 15.625 ... is a geometric sequence .
Option 3)9.1,9.2,9.3,9.4....
Common ratio=

=
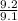
=
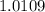
Common ratio=
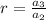
=
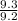
=
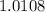
Common ratio=

=
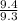
=
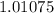
Since the common ratios are not equal .
Thus 9.1,9.2,9.3,9.4.... is not a geometric sequence .
Option 4) 8,0.8,0.08,0.008 ....
Common ratio=

=
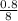
=
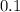
Common ratio=
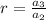
=
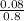
=
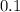
Common ratio=

=
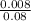
=
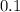
Since the common ratios are equal .
Thus -18,0.8,0.08,0.008 .... is a geometric sequence .
Option 5) 4,-4,-12,-20...
Common ratio=

=
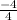
=
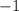
Common ratio=
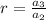
=
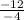
=
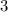
Common ratio=

=
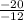
=
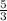
Since the common ratios are not equal .
Thus 4,-4,-12,-20... is not a geometric sequence .