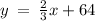Question

Mario compared the slope of the function graphed below to the slope of the linear function that has an x-intercept of 1 and a y-intercept of -2.

Find the slope of both lines. In the answer box give the slope of the steeper line.

Answer 1

The linear equation in the slope-intercept form will be:

`y\:=\:(2)/(3)x+64`

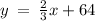
Explanation:

From the line graph, taking two points

• (21, 78)

• (27, 82)

Finding the slope between (21, 78) and (27, 82)

`\mathrm{Slope}=(y_2-y_1)/(x_2-x_1)`

`\left(x_1,\:y_1\right)=\left(21,\:78\right),\:\left(x_2,\:y_2\right)=\left(27,\:82\right)`

`m=(82-78)/(27-21)`

`m=(2)/(3)`

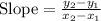
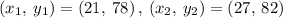
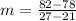
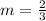
We know that the slope-intercept of the line equation is

`y = mx+b`

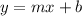
where m is the slope and b is the y-intercept

substituting (21, 78) and m = 2/3 in the slope-intercept of the line

`y = mx+b`

`78=(2)/(3)\left(21\right)+b`

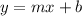
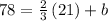
switch sides

`(2)/(3)\left(21\right)+b=78`

`14+b=78`

`b=64`

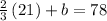
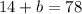
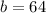
substituting b = 64 and m = 2/3 in the slope-intercept of the line

`y = mx+b`

`y\:=\:(2)/(3)x+64`

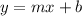
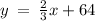
Therefore, the linear equation in the slope-intercept form will be:

`y\:=\:(2)/(3)x+64`