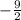Answer:
The slope of the parallel line to the given line is

The equation of the parallel line to the given line and passes through the given point is y + 4 =
 (x + 2)
(x + 2)
The y-intercept of the parallel line to the given line and passes through the given point is
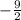
Explanation:
- The rule of the slope of the line that passes through points (x1, y1) and (x2, y2) is m =
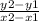
- The point-slope form of the linear equation is y - y1 = m(x - x1), where m is the slope and (x1, y1) is a point on the line
- The slope-intercept form of the linear equation is y = m x + b, where m is the slope and b is the y-intercept
- Parallel lines have the same slopes and different y-intercepts
In the given figure
∵ The given line passes through points (2, 6) and (-6, 8)
∴ x1 = 2 and y1 = 6
∴ x2 = -6 and y2 = 8
→ Substitute them in the rule of the slope above to find it
∵ m =
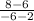 =
=
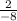 =
=

∴ The slope of the given line is

∵ Parallel lines have the same slopes
∴ The slope of the parallel line to the given line is

∵ The parallel line passes through the point (-2, -4)
∴ x1 = -2 and y1 = -4
∵ m =

→ Substitute them in the point-slope form above
∵ y - (-4) =
 (x - (-2))
(x - (-2))
∴ y + 4 =
 (x + 2)
(x + 2)
∴ The equation of the parallel line to the given line and passes through
the given point is y + 4 =
 (x + 2)
(x + 2)
∵ m =

→ Substitute it in the slope-intercept form above
∴ y =
 x + b
x + b
→ To find b substitute x by -2 and y by -4 (coordinates the given point)
∵ -4 =
 (-2) + b
(-2) + b
∴ -4 =
 + b
+ b
→ Subtract
 from both sides
from both sides
∴
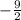 = b
= b
∵ b is the y-intercept
∴ The y-intercept of the parallel line to the given line and passes
through the given point is