Question

Please halpp
i need this done soon

Answer 1

B. x² - 3x - 40

Explanation:

The factored form of quadratic function:

`y=a(x-x_1)(x-x_2)`

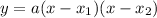
where x₁ and x₂ are the roots of the quadratic function and the solutions of the corresponding quadratic equation.

We have x₁ = 8 and x₂ = -5. Substitute:

`y=a(x-8)(x-(-5))`

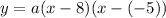
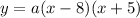`y=a(x-8)(x+5)` use FOIL: (a + b)(c + d) = ac + ad + bc + bd

`y=a[(x)(x)+(x)(5)+(-8)(x)+(-8)(5)]`

`y=a(x^2+5x-8x-40)`

`y=a(x^2-3x-40)`

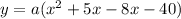
For a = 1 we have the equation:

B. x² - 3x - 40