Question

PLEASE PLEASE HELP

The half-life of a radioactive isotope is the time it takes for a quantity of the isotope to be reduced to half its initial mass. Starting with 185 grams of a radioactive isotope, how much will be left after 6 half-lives?
Use the calculator provided and round your answer to the nearest gram.

Answer 1

Approximately 3 grams left.

Explanation:

We will utilize the standard form of an exponential function, given by:

`f(t)=a(r)^t`

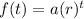
In the case of half-life, our rate r will be 1/2. This is because 1/2 or 50% will be left after t half-lives.

Our initial amount a is 185 grams.

So, by substitution, we have:

`\displaystyle f(t)=185\big((1)/(2)\big)^t`

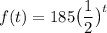
Where f(t) denotes the amount of grams left after t half-lives.

We want to find the amount left after 6 half-lives. Therefore, t = 6. Then using our function, we acquire:

`\displaystyle f(6)=185\big((1)/(2)\big)^6`

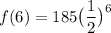
Evaluate:

`\displaystyle f(6)=185\big((1)/(64)\big)\approx2.89\approx 3`

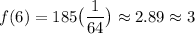
So, after six half-lives, there will be approximately 3 grams left.