11. Divide both sides by 2:
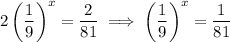
The solution has to be
 because
because
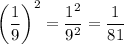
12. Divide both sides by 2:
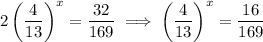
On the right side we have two perfect squares:
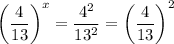
so again the answer is
 .
.
14. Divide both sides by 8:
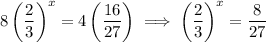
On the right we have perfect cubes:
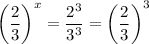
so
 .
.
15.

We could divide both sides by 2/5 (or multiply both sides by 5/2, as the writing on your paper suggests). Then

The right side has two perfect squares:
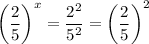
so that
 .
.
Another way to do this is to rewrite the left side as

Meanwhile, on the right we have two perfect cubes:

so that
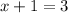 , or
, or
 , as before.
, as before.