Question

4. Gasoline, which we will represent as having the properties of isooctane, C8H18, is consumed by an idling automobile engine at a rate of 1 gal/h. CO is a toxic air pollutant formed from the incomplete combustion of the gasoline. An air monitor in the garage where work is being done on an engine detects an accumulation of CO. What does this information imply about the gasoline-to-air ratio being fed to the engine? If we assume that gasoline has properties of isooctane, estimate the feed rate (mol/h) of air for 10% excess oxygen fed to the engine. The MW and density of isooctane is 114.23 g/mol and 691.87 kg/m3, respectively. Air contains 21 mol % O2. (hint: balance the stoichiometric equation to determine the theoretical number of moles of O2, C8H18 + n O2 = n H2O + n CO2).

Answer 1

Step-by-step explanation:

Volume of fuel used per hour = 1 gal /h

1 gal =
`0.0037 m^3`

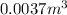
Mass of the fuel =
`Volume* Density =0.0037 m^3* 691.87 kg/m^3=2.5599 kg = 2559.9 g`

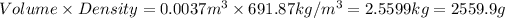
Moles of isoactane:
`(2559.9 g)/(114 g/mol)=22.41 moles`

`2C_8H_(18)+25O_2\rightarrow 18H_2O+16CO_2`

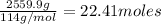
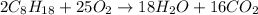
2 moles of iso-octane react with 25 moles of oxygen then 22.41 moles of iso-octane will react with :
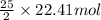`(25)/(2)* 22.41 mol` of oxygen that is 280.125 moles.

We are given that Air contains 21 mole % of oxygen:

`21=\frac{280.125 moles}{\text{moles of Air}}* 100`

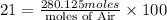
Moles of Air =1333.92 moles

Feed rate of the air = 1333.92/h

Ratio of gasoline to air being fed to engine:

`\frac{\text{rate of fuel consumed}}{\text{Feed rate of air}}=(22.41 /h)/(1333.92 mol/h)=0.000182`

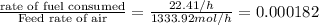
If the ratio gasoline to air is less than or equal to 0.000749 than no accumulation of CO will be there in an engine.

The CO got accumulated in the engine which means that ratio of gasoline to air is greater than the 0.000749.