Question

I need it step by step please​

Answer 1

The equation of the line passes through (3, -2) and parallel to the given line:

• 
  `y = 3x-11`

The equation of the line passes through (3, -2) and perpendicular to the given line:

• 
  `y=-(1)/(3)x-1`

Explanation:

Given the points on the graph line

(2, -1)

(1, -4)

Finding the slope between (2, -1) and (1, -4)

`\mathrm{Slope}=(y_2-y_1)/(x_2-x_1)`

`\left(x_1,\:y_1\right)=\left(2,\:-1\right),\:\left(x_2,\:y_2\right)=\left(1,\:-4\right)`

`m=(-4-\left(-1\right))/(1-2)`

`m=3`

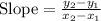
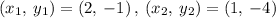
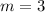
Equation of the line passes through (3, -2) and parallel to the given line.

We know that parallel lines have the same slope.

so the equation of the line parallel to the given line = 3

Thus, using the point-slope form of the line equation

`y-y_1=m\left(x-x_1\right)`

where m is the slope of the line and (x₁, y₁) is the point

substituting the values m = 3 and the point (3, -2)

`y - (-2) = 3(x-3)`

`y+2 = 3x-9`

`y = 3x-9-2`

`y = 3x-11`

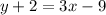
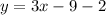
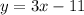
Therefore, the equation of the line passes through (3, -2) and parallel to the given line:

`y = 3x-11`

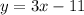
The equation of the line passes through (3, -2) and perpendicular to the given line

We know that a line perpendicular to another line contains a slope that is the negative reciprocal of the slope of the other line, such as:

slope = m = 3

perpendicular slope = – 1/m = -1/3 = -1/3

Therefore, substituting the values of perpendicular slope = -1/3 and the point (3, -2) in the point-slope form of the line equation

`y-\left(-2\right)=-(1)/(3)\left(x-3\right)`

`y+2=-(1)/(3)\left(x-3\right)`

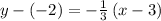
subtract 2 from both sides

`y+2-2=-(1)/(3)\left(x-3\right)-2`

`y=-(1)/(3)x-1`

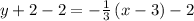
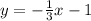
Therefore, the equation of the line passes through (3, -2) and perpendicular to the given line:

`y=-(1)/(3)x-1`

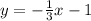
Conclusion:

The equation of the line passes through (3, -2) and parallel to the given line:

• 
  `y = 3x-11`

The equation of the line passes through (3, -2) and perpendicular to the given line:

• 
  `y=-(1)/(3)x-1`