Question

2v^2-12v-29=3

Find the Vertex Form and Vertex

Answer 1

see explanation

Explanation:

The equation of a parabola in vertex form is

y = a(x - h)² + k

where (h, k) are the coordinates of the vertex and a is a multiplier

given a quadratic in standard form : y = ax² + bx + c : a ≠ 0

Then the x- coordinate of the vertex is

`x_(vertex)` = -
`(b)/(2a)`

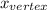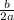
Rearrange 2v² - 12v - 29 = 3 into standard form ( subtract 3 from both sides )

2v² - 12v - 32 = 0 ← in standard form

with a = 2, b = - 12, hence

`x_(vertex)` = -
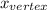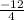`(-12)/(4)` = 3

substitute x = 3 into the equation for corresponding value of y

y = 2(3)² - 12(3) - 32 = 18 - 36 - 32 = - 50

vertex = (3, - 50)

y = 2(v- 3)² - 50 ← in vertex form

Answer 2

The vertex form is
`2(v-3)^(2)-50`

The vertex is (3 , -50)

Explanation:

∵
`2v^(2)-12v-29 = 3` ⇒
`2v^(2)-12v-29-3=2v^(2)-12v-32=a(v+b)^(2)+c`, where a , b and c are constant

`2v^(2)-12v-32=a(v^(2)+2bv+b^(2))+c`

`2v^(2)-12v-32=av^(2)+2abv+ab^(2)+c`⇒ compare the two sides

a = 2

2ab = -12 ⇒ 2(2)b = -12 ⇒ 4b = -12 ⇒ b = -3

`ab^(2)+c=-32` ⇒
`2(-3)^(2)+c=-32`

2 × 9 + c = -32 ⇒ c = -32 -18 = -50

∴ The vertex form is
`2(v-3)^(2)-50`

∴ The vertex is (3 , -50)