Question

Which of the following is the correct classification of ∆mnp if m<m=35° and m<p=47°?​

Answer 1

D. Obtuse.

Explanation:

We are told that in
`\Delta MNP`
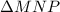
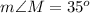`m\angle M=35^o` and
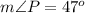`m\angle P=47^o`. We are asked to classify our given
`\Delta MNP`.

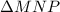We can see that angle M and angle P are acute angles as their measure is less than 90 degrees.

Let us find the measure of angle P using angle sum property of triangles, which states that sum of interior angles of a triangle is 180 degrees.

So we can set an equation as:

`m\angle M+m\angle P+m\angle N=180^o`

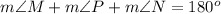
Upon substituting our given values we will get,

`35^o+47^o+m\angle N=180^o`

`82^o+m\angle N=180^o`

`82^o-82^o+m\angle N=180^o-82^o`

`m\angle N=98^o`

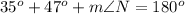
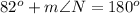
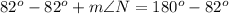
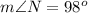
As measure of angle N is 98 degrees, so angle N is an obtuse angle.

Since a triangle having an angle that measures more than 90 degrees is called an obtuse triangle, therefore,
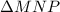`\Delta MNP` is an obtuse triangle and option D is the correct choice.