Question

Given f(x)=sec x and g(x)=arcsin x, what is f(g(x))?

Answer choices:

A. Sqrt 1-x^2 / 1-x^2

B. Sqrt x^2+1 / x^2+1

C. Sqrt 1-x^2

D. Sqrt x^2+1

Answer 1

Option 1 is correct.

Explanation:

Given two functions f(x)=sec x and g(x)=arcsin x

we have to find f(g(x)).

f(g(x))=sec(arcsinx)=sec A where A=arc(sinx)

As, A=arc(sinx)

⇒
`x=sin A=(P)/(H)`

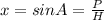
⇒ P=x and H=1 gives
`B=√(1-x^2)`

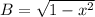
hence,
`sec A=(H)/(B)=(1)/(√(1-x^2))`

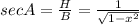
hence,
`f(g(x))=(1)/(√(1-x^2))`

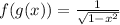
Rationalizing, we get

`f(g(x))=(√(1-x^2))/(1-x^2)`

Option 1 is correct.