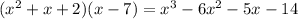Answer:
The remainder is zero
Explanation:
To find the remainder we will use the long division
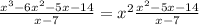 ⇒(1)
⇒(1)
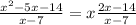 ⇒(2)
⇒(2)
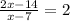 ⇒(3)
⇒(3)
From (1) , (2) and (3)
The quotient of the long division is
 and no remainder
and no remainder
So the remainder is zero
* If you want to check your answer Multiply the quotient by the divisor