Answer:
b = ∛16 ≈ 2.520
Explanation:
The area bounded by y=x^2 and y=b is given by the integral ...
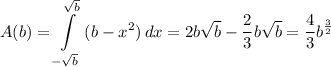
For b = 4,
A(4) = (4/3)4^(3/2) = 32/3
We want to find b such that the area is half that, or ...
A(b) = (1/2)(32/3) = 16/3
So, we're solving the equation ...
16/3 = 4/3b^(3/2)
4 = b^(3/2) . . . . . . . . . . . . . . multiply by 3/4
4^(2/3) = b = ∛16 . . . . . . . . raise to the 3/2 power