Question

PLS HELP 50PTS

An architect is planning to make two triangular prisms out of iron. The architect will use ∆PQR for the bases of one prism and ∆XYZ for the bases of the other prism.




Suppose the height of the prism made by ∆PQR is 15 inches. What is the volume of the prism made by ∆PQR? Remember to show your work.


Suppose the volume of the prism made by ∆PQR is 7776 〖"in" 〗^3. What is the volume of the prism made by ∆XYZ? Remember to show your work.

Answer 1

A.
`\text{Volume of the prism made by }\Delta PQR =12960\text{ inch}^3`

B.
`\text{Volume of prism made by }\Delta XYZ=2304\text{ in}^3`

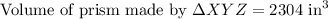
Explanation:

We have been given bases of two triangular prisms.

A. Since we know that volume of triangular prism is base area of the prism times height of the prism.

`\text{Volume of triangular prism}=\text{Base area* Height of the prism}`

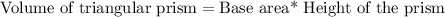
Since base of our given prism is right triangle, so area of the base of prism will be:
`(1)/(2)* 48\text{ inch}* 36\text{ inch}`

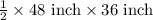
Upon substituting our given values in volume formula we will get,

`\text{Volume of the prism made by }\Delta PQR =(1)/(2)* 48 \text{ inch}* 36 \text{ inch}* 15\text{ inch}`

`\text{Volume of the prism made by }\Delta PQR =(1)/(2)* 48* 36* 15\text{ inch}^3`

`\text{Volume of the prism made by }\Delta PQR =24* 36* 15\text{ inch}^3`

`\text{Volume of the prism made by }\Delta PQR =12960\text{ inch}^3`

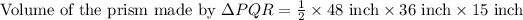
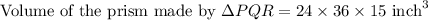
Therefore, volume of the prism made by triangle PQR is 12960 cubic inches.

B. Let us assume that both prism are similar, so we can use proportions to solve for the volume of triangle XYZ.

Let us find the proportion between the sides of both triangles.

`\frac{\text{Side of triangle XYZ}}{\text{Side of triangle PQR}}=(24)/(36)`

`\frac{\text{Side of triangle XYZ}}{\text{Side of triangle PQR}}=(12*2)/(12*3)`

`\frac{\text{Side of triangle XYZ}}{\text{Side of triangle PQR}}=(2)/(3)`

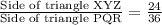
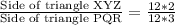
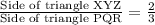
Since for volume of triangular prism we multiply base area and height of the prism, this means we will have to multiply the proportion of each side length 3 times to find the proportion of volumes between our both prism.

So we can set proportion for volume of both prisms as:

`\frac{\text{Volume of prism made by triangle XYZ}}{\text{Volume of prism made by triangle PQR}}=((2)/(3))^3`

`\frac{\text{Volume of prism made by triangle XYZ}}{\text{Volume of prism made by triangle PQR}}=(8)/(27)`

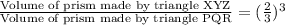
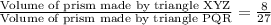
Upon substituting volume of prism made by triangle PQR we will get,

`\frac{\text{Volume of prism made by triangle XYZ}}{7776\text{in}^3}=(8)/(27)`

Let us multiply both sides of our equation by 7776 cubic inches.

`\frac{\text{Volume of prism made by triangle XYZ}}{7776\text{ in}^3}*7776\text{ in}^3=(8)/(27)*7776\text{ in}^3`

`\text{Volume of prism made by triangle XYZ}=8*288\text{ in}^3`

`\text{Volume of prism made by triangle XYZ}=2304\text{ in}^3`

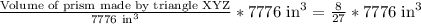
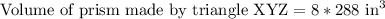
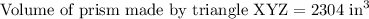
Therefore, the volume of prism made by triangle XYZ is 2304 cubic inches.

Answer 2

Answer:Volume of triangular prism = Base area* Height of the prism

Volume of the prism made by APQR = x 48 x 36 x 15 inch³

Volume of the prism made by APQR = 24 x 36 x 15 inch³

Volume of the prism made by APQR =12960in³