Answer:
The required system of equations is,
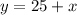
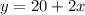 .
.
Explanation:
Let the number of hours spent by Lucy = x
It is given that,
Local zoo charges initial fee $25 and per hour fee $1.
So, the equation representing the cost if Lucy stays 'x' hours is,
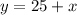 in dollars.
in dollars.
Also, Local citcus charges initial fee $20 and per hour fee $2.
So, the equation representing the cost if Lucy stays 'x' hours is,
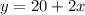 in dollars.
in dollars.
Since, the objective function for the situation is to 'minimize the cost'.
So, upon solving the equations, we will get the minimum value as,
25+x = 20+2x
i.e. x= 5
Thus,
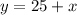 implies
implies
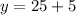 i.e. y= $30
i.e. y= $30
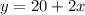 implies
implies
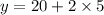 i.e.
i.e.
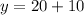 i.e. y= $30
i.e. y= $30
Thus, the minimum value is $30.
The required system of equations is,
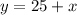
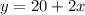 .
.