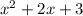Question

What is the quotient (x^3+3x^2+5x+3)/(x+1)

Answer 1

observe that

x³ + 3x² + 5x + 3 = x³ + 3x² + 3x + 1 + 2x + 2

= (x + 1)³ + 2(x + 1)

= (x + 1)((x + 1)² + 2)

= (x + 1)(x² + 2x + 3)

so the quotient is

x² + 2x + 3

Answer 2

`x^(2) +2x+3`

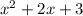
Explanation:

Wehn you are faced with the next division you have to factorize the polynomial function:

`(x^(3)+3x^(2)+5x+3)/(x+1)`

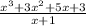
Now you have to separate it in two factors, one of them being (x+1) so you can after eliminate it:

`((x+1)(x^(2)+2x+3))/(x+1)`

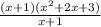
And now you just eliminate the same binomials from both sides of the division and you´d end up with the next division:

`(x^(2)+2x+3)/(1)`

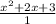
And the answer to the division is:

`x^(2) +2x+3`