Question

Question in picture

A) 16/63

B)-16/63

C) 63/16

D) -63/16

Answer 1

ANSWER

`\tan(x + y) = - (63)/(16)`


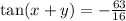
EXPLANATION


We were given that,


`\csc(x) = (5)/(3)`

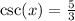
This implies that,


`\sin(x) = (3)/(5)`

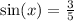
We use the Pythagorean identity


`\sin^(2) (x) + \cos^(2) (x)= 1`
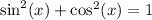
to get,



`\cos(x) = \sqrt{1 - ( { (3)/(5) })^(2)} = (4)/(5)`


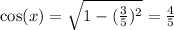
We were also given that,



`\cos(y) = (5)/(13)`

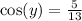
This means that,



`\sin(y) = \sqrt{1 - {( (5)/(13)) }^(2) } = (12)/(13)`

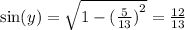
This is because,



`0 < \: x \: < (\pi)/(2)`



`0 < \: y \: < (\pi)/(2)`

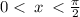
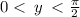
This angles are in the first quadrant so we pick the positive values.


`\tan(x + y) = ( \sin(x + y) )/( \cos(x + y) )`



`\tan(x + y) = ( \sin(x ) \cos(y) + \sin(y) \cos(x) )/( \cos(x) \cos(y) - \sin(x) \sin(y) )`




`\tan(x + y) = ( (3)/(5) * (5)/(13) + (12)/(13) * (4)/(5) )/( (4)/(5) * (5)/(13) - (3)/(5) * (12)/(13) )`




`\tan(x + y) = - (63)/(16)`

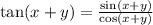
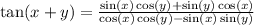
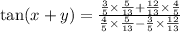
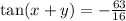
The correct answer is D