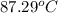Question

A 52.0 g of Copper (specific heat=0.0923cal/gC) at 25.0C is warmed by the addition of 299 calories of energy. find the final temperature of copper​

Answer 1

Answer : The final temperature of the copper is,
`87.29^oC`

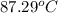
Solution :

Formula used :

`Q=m* c* \Delta T=m* c* (T_(final)-T_(initial))`

where,

Q = heat gained = 299 cal

m = mass of copper = 52 g

c = specific heat of copper =
`0.0923cal/g^oC`

`\Delta T=\text{Change in temperature}`

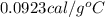
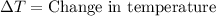
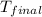`T_(final)` = final temperature = ?

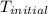`T_(initial)` = initial temperature =
`25^oC`

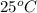
Now put all the given values in the above formula, we get the final temperature of copper.

`299cal=52g* 0.0923cal/g^oC* (T_(final)-25^oC)`

`T_(final)=87.29^oC`

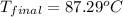
Therefore, the final temperature of the copper is,
`87.29^oC`