Question

A group of retailers will buy 104 televisions from a wholesaler if the price is $300 and 144 if the price is $250. The wholesaler is willing to supply 88 if the price is $225 and 168 if the price is $315. Assuming the resulting supply and demand functions are linear, find the equilibrium point for the market.

(q,p):

Answer 1

The required equilibrium point is (356.538,58.769)

Explanation:

The two points on your demand equation are : (300,104) and (250,144)

Take the number of televisions on y-axis and the price of the television on x-axis

Now, to find the demand equation for the given problem, find slope using the above two points :

`\implies Slope=(144-104)/(250-300)\\\\\implies Slope = -(4)/(5)=-0.8`

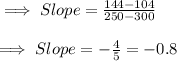
And the y - intercept is : y = -0.8x + b

Find the value of b using any point. Let us take (250,144)

144 = -0.8 × 250 + b

⇒ b = 144 + 200

⇒ b = 344

So, the y-intercept is : y = -0.8x + 344

The two points on your supply equation are : (225,88) and (315,68)

Take the number of televisions on y-axis and the price of the television on x-axis

Now, to find the supply equation for the given problem, find slope using the above two points :

`\implies Slope=(68-88)/(315-225)\\\\\implies Slope = -(2)/(9)`

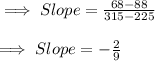
And the y - intercept is :

`y = -(2)/(9)x + b`

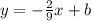
Find the value of b using any point. Let us take (225,88)

`88 = -(2)/(9)* 225 + b\\\\\implies b = 88 + 50\\\\\implies b = 138`

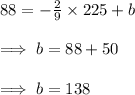
So, the y-intercept is :

`y = -(2)/(9)x + 138`

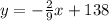
To find the equilibrium point, solve both the y-intercepts of the demand equation and the supply equation :

⇒ x = 356.538 and y = 58.769

Hence, the required equilibrium point is (356.538,58.769)