Question

A velocity selector is built with a magnetic field of magnitude 5.2 T and an electric field of strength 4.6 × 10 ^4 N/C. The directions of the two fields are perpendicular to each other. At what speed will electrons pass through the selector without deflection? Let the charge of an electron q = −1.6 × 10 ^ −19

A.8.8 × 10^3 m/s
B 2.4 × 10 ^ - 15 m/s
C 7.9 × 10 ^ 13 m/s
D 2.0 x 10 ^ 4 m/s

Answer 1

Answer: 8.8×10³ m/s

Step-by-step explanation:

When an electron enters electric field and magnetic field, Lorentz force acts on it which displaces it path.

Lorentz force is given by: F = q E + q v×B

For electron to not deflect from its path, this force must be zero which means the speed of the electron should be:

`v =(E)/(B)\\ \Rightarrow v = (4.6* 10^4N/C)/(5.2 T) = 8.8* 10^3 m/s`

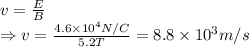
Thus, the electrons would pass through the selector without deflection at the speed of 8.8 × 10³ m/s.

Answer 2

Option A that is 8.8 × 10^3 m/s

Explanation

The magnetic field B is defined from the Lorentz Force Law, and specifically from the magnetic force on a moving charge. It says

Field-strength = BVqsinΔ

v = E/B

Since field are perpendicular so sin90 = 1

v = 4.6/10^4 / 5.2

v = 8846.15 m /s

The speed at which electrons pass through the selector without deflection = 8846.15 m /s