Question

A 0.54 kg particle has a speed of8.0 m/s at point A and kineticenergy of 7.5 J at point B.

(a) What is its kinetic energy at A? J
(b) What is its speed at point B?m/s
(c) What is the total work done on the particle as it moves fromA to B? J

Answer 1

a) The translational kinetic energy of the particle at point A is 17.28 joules.

b) The speed of the particle at point B is approximately 5.270 meters per second.

c) The total work done on the particle as it moves from A to B is - 9.78 joules.

Step-by-step explanation:

Let be this particle a conservative system, that is, that non-conservative forces (i.e. friction, viscosity) are negligible.

a) The translational kinetic energy of the particle (
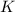`K`), measured in joules, is determined by the following formula:

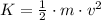`K = (1)/(2)\cdot m \cdot v^(2)` (1)

Where:

`m` - Mass, measured in kilograms.

`v` - Speed, measured in meters per second.

If we know that
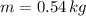`m = 0.54\,kg` and
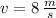`v = 8\,(m)/(s)`, the translational kinetic energy at point A is:

`K = (1)/(2)\cdot (0.54\,kg)\cdot \left(8\,(m)/(s) \right)^(2)`

`K = 17.28\,J`

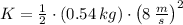
The translational kinetic energy of the particle at point A is 17.28 joules.

b) The speed of the particle is clear in (1):

`v = \sqrt{(2\cdot K)/(m) }`

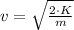
If we know that
`K = 7.5\,J` and
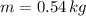`m = 0.54\,kg`, then the speed of the particle at point B:

`v = \sqrt{(2\cdot (7.5\,J))/(0.54\,kg) }`

`v\approx 5.270\,(m)/(s)`

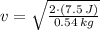
The speed of the particle at point B is approximately 5.270 meters per second.

c) According to the Work-Energy Theorem, the total work done on the particle as it moves from A to B (
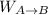`W_(A\rightarrow B)`), measured in joules, is equal to the change in the translational kinetic energy of the particle. That is:

`W_(A\rightarrow B) = K_(B)-K_(A)` (2)

If we know that
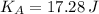`K_(A) = 17.28\,J` and
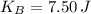`K_(B) = 7.50\,J`, then the change in the translational kinetic energy of the particle is:

`W_(A\rightarrow B) = 7.50\,J-17.28\,J`

`W_(A\rightarrow B) = -9.78\,J`

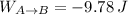
The total work done on the particle as it moves from A to B is - 9.78 joules.