Answer:
The ship is 25.54 miles far from the light house.
Explanation:
It is given that A boat travels 26 miles East from a lighthouse then changes direction traveling 15° South of West for 13 miles that is :
BC=c, BA=26 miles and CA=13miles
Then, applying the cosine formula in ΔABC, we get
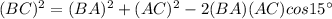
⇒
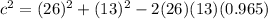
⇒
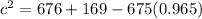
⇒

⇒
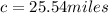
Therefore, the ship is 25.54 miles far from the light house.