Answer:
Option 1. Down 2, right pi over 3.
Explanation:
In a given function f(x) = -7 sin(3x+π)-2 we have to find the shift horizontally and vertically.
As we know in a sine equation represented as y = a sin(bx+c) +d
Amplitude of the wave = a
Period =
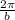
Horizontal shift =

Vertical shift = d
From the given sine function we can get the following values
a = 7
period =
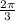
horizontal shift =
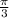 in the right side since it's with positive sign.
in the right side since it's with positive sign.
Vertical shift = -2 down with 2 ( since it's with negative sign)
Therefore Option 1 is the correct one.