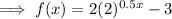Question

Pls see below very short question

Answer 1

`f(x)=2(2)^(0.5x)-3`

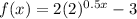
Explanation:

Parent function:

`g(x)=2^x`

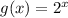
Properties of the given parent function:

• y-intercept at (0, 1)
• horizontal asymptote at y = 0
• As x → -∞, y → 0
• As x → ∞, y → ∞

Given form of function f(x):

`f(x)=a(b)^(kx)+c`

If the parent function is
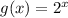`g(x)=2^x` then b = 2:

`\implies f(x)=a(2)^(kx)+c`

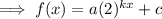
From inspection of the graphed function f(x):

• y-intercept at (0, -1)
• horizontal asymptote at y = -3

Therefore, the y-intercept has shifted 2 units down, yet the asymptote has shifted 3 units down. This implies that there has been a vertical shift of 3 units down and a vertical stretch.

The vertical shift is denoted by the variable "c" so c = -3:

`\implies f(x)=a(2)^(kx)-3`

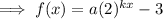
The vertical stretch is denoted by the variable "a". To find value of a, substitute the point of the y-intercept into the equation:

`\begin{aligned}f(0) & = -1\\\implies a(2)^(k * 0)-3 & =-1\\a-3 & = -1\\a-3+3 & = -1+3\\a & = 2\end{aligned}`

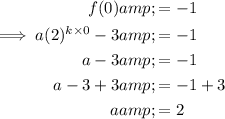
Therefore, as a = 2:

`\implies f(x)=2(2)^(kx)-3`

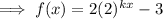
From inspection of the given graph, the curve passes through point (4, 5). Substitute this point into the equation to find the value of k:

`\begin{aligned}f(4) & = 5\\\implies 2(2)^(4k)-3 & =5\\2(2)^(4k)& =8\\(2)^(4k)& =4\\(2)^(4k)& =2^2\\4k & = 2\\k & = 0.5\end{aligned}`

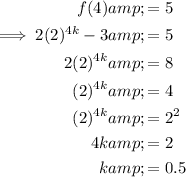
Therefore, the equation of the function f(x) is:

`\implies f(x)=2(2)^(0.5x)-3`