Answer:
see explanation
Explanation:
Since x = - 5 is a root of the equation then substituting x = - 5 into the equation allows q to be found
3(- 5)² + 7(- 5) - q = 0
75 - 35 - q = 0
40 - q = 0 ( subtract 40 from both sides )
- q = - 40 ⇒ q = 40
The equation can now be written as
3x² + 7x - 40 = 0
Factorise to find the other root
Consider the factors of the product of the x² term and the constant term which sum to give the coefficient of the x- term
product = 3 × - 40 = - 120 and sum = + 7
The factors are + 15 and - 8
Express the x- term using these factors
3x² + 15x - 8x - 40 = 0 ( factor the first/second and third/fourth terms )
3x(x + 5) - 8(x + 5) = 0 ← factor out (x + 5)
(x + 5)(3x - 8) = 0
Equate each factor to zero and solve for x
x + 5 = 0 ⇒ x = - 5 ← root already known
3x - 8 = 0 ⇒ x =
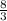 ← the other root
← the other root