Question

*The sum of two numbers is 400. If the first number were decreased by 20% and the second number were decreased by 15%, then the sum would be 68 less. Find the numbers after decreasing.

Answer 1

128 and 204

Step-by-step explanation (note, it is not the shortest way):

- if the initial value of the 1st number is 'x' and of the 2d number is 'y', then after decreasing their values is 0.8x and 0.85y. The phrase 'then the sum would be 68 less' means the sum after decreasing is 400-68=332.

- Using this it is possible to make up the system of two equations then resolve it:

`\left \{ {{x+y=400} \atop {0.8x+0.85y=332}} \right. \ => \ \left \{ {{x=160} \atop {y=240}} \right.`

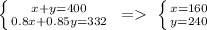
- before decreasing x=160 and y=240. After decreasing x=160*0.8=128 and y=0.85*240=204.