Hello!
The answer is: There are 9 packets of 10-battery packets.
Why?
Let's make the calculations:
Let y b the 6-battery packet and z be the 10-battery packet.
We must construct the following to equations using the statement information: There is a stock of 25 packets and there is a total of 186 batteries formed by 6-battery packets and 10-battery packers
So, the first equation will be:

The second equation will be:
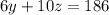
From the first equation we have:
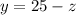
Substituting y in the second equation we have:
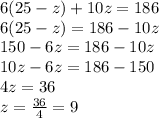
So, there are 9 packets of 10-battery packets.
By substituting z in the first equation we will know the number of 6-battery packets in stock

So, there are 16 packets of 6-battery packets.
Have a nice day!