Question

The loudness, L, of a sound (measured in decibels, dB) is inversely proportional to the square of the distance, d

from the source of the sound.
A person 14 feet from a jetski, it is 85 decibels loud.
How loud is the jetski when the person is 41 feet away? (Round to 3 decimal places.)
dB

Answer 1

L = 9.91 decibels.

Explanation:

The loudness, L is inversely proportional to the square of the distance, d, from the source of the sound.

i.e L
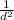
`\alpha`
`(1)/(d^(2) )`

L =
`(k)/(d^(2) )`

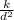
Where k is the constant of proportionality.

When d = 14 feet and L = 85 dB, then;

k = L x
`d^(2)`

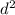
= 85 x
`(14)^(2)`

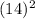
= 16660

k = 16660

L =
`(16660)/(d^(2) )`

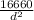
Thus, when d = 41 feet, then;

L =
`(16660)/((41)^(2) )`

=
`(16660)/(1681)`

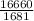
= 9.91077

L = 9.91 dB

The loudness is 9.91 decibels.