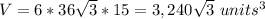Question

A right prism has height 15 and bases that are regular hexagons with sides of length 12. Find the volume of the prism.

Answer 1

The volume of the hexagon is equal to
`3,240√(3)\ units^(3)`

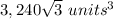
Explanation:

we know that

The volume of the prism is equal to

`V=BH`

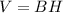
where

B is the area of the base of the prism

H is the height of the prism

we have

`H=15\ units`

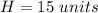
To find the area of the base (hexagon) calculate the area of one equilateral triangle and then multiply by 6

`A=(1)/(2)bh`

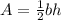
we have

`b=12\ units`

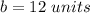
Applying Pythagoras theorem calculate the height of the triangle

`h^(2)=12^(2)-6^(2)`

`h^(2)=144-36`

`h^(2)=108`

`h=√(108)=6√(3)\ units`

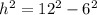
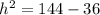
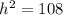
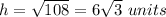
substitute

The area of one triangle is equal to

`A=(1)/(2)(12)(6√(3))`

`A=36√(3)\ units^(2)`

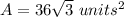
Find the volume of the prism

`V=6*36√(3)*15=3,240√(3)\ units^(3)`