Answer: There are 7 roots for the polynomial function.
Explanation:
Since we have given that
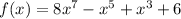
We need to find the number of roots exist for the polynomial.
As we know that
Number of roots = Highest degree of the polynomial.
So, the number of roots = 7
Hence, there are 7 roots for the polynomial function.