Taking
 .
.
- Worker A: 476 N.
- Worker B: 324 N.
Step-by-step explanation
Worker A:
Consider the pipe as a level. Worker A will supply the effort, and worker B will act as the fulcrum.
- Worker A applies an upward force 4.2 meters away from the fulcrum.
- The weight of the pipe acts at the center of its mass, which is 2.5 meters away from the fulcrum. The level is class two.
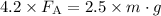
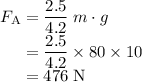
Worker B:
Again, consider the pipe as a level. Worker B will now supply the effort, and worker A will act as the fulcrum.
- Worker B applied an upward force
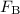 4.2 meters away on the left-hand side of the fulcrum.
4.2 meters away on the left-hand side of the fulcrum.
The weight of the pipe acts at two positions.
 of the pipe's mass acts downwards between worker A and B. That
of the pipe's mass acts downwards between worker A and B. That
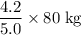 of mass will act downward at its center of mass
of mass will act downward at its center of mass
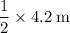 away on the left-hand side of the fulcrum.
away on the left-hand side of the fulcrum.
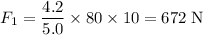
 of the pipe's mass acts downwards to the right of worker B. That
of the pipe's mass acts downwards to the right of worker B. That
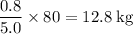 of mass will act also downward at its center of mass
of mass will act also downward at its center of mass
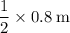 away on the right-hand side of the fulcrum.
away on the right-hand side of the fulcrum.
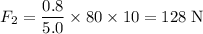
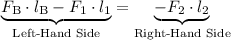 for the pipe to balance. Therefore:
for the pipe to balance. Therefore:
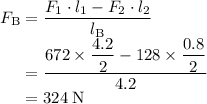 .
.
Make sure that
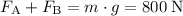 .
.