Solution
In this question we have given
Charge,
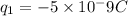
Charge,
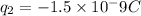
Charge,
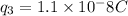
Distance between Charge
 and
and
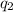 ,d=43cm=.43m
,d=43cm=.43m
Let the distance between charge
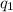 and
and
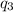 , is x
, is x
Therefore,distance between charge
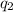 and
and
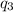 , will be=(.43-x)
, will be=(.43-x)
We know By Couloms law, Force on Charge
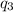 due to charge
due to charge
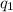 which are seperated by distance d is given by following equation
which are seperated by distance d is given by following equation
 ............(1)
............(1)
Here, K=
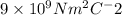
Therefore,
Force on Charge
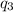 due to charge
due to charge
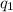
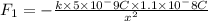 ..(2)
..(2)
Similarly,
Force on Charge
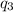 due to charge
due to charge
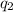
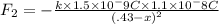 ..(2)
..(2)
In equilibrium condition,


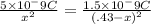
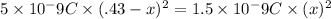
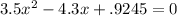
on solving we get
x=.277m
0r x=28cm
The distance between charge
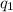 and
and
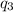 , is 28cm
, is 28cm